8.10 Volume with Disc Method: Revolving Around Other Axes
3 min read•june 18, 2024
AP Calculus AB/BC ♾️
279 resourcesSee Units
Volumes of solids of revolution around a horizontal or vertical line in the plane can be calculated by using the disc method and definite integrals. The disc method involves approximating the solid by a large number of thin circular discs, which are stacked along the axis of revolution to form the solid. The volume of each disc can be calculated using the formula for the area of a circle, and the sum of the volumes of all the discs can be used to approximate the volume of the solid. 💿
To use the disc method, the solid must first be defined by a function that describes the cross-sectional shape of the solid at any point along the axis of revolution. Then, the area of each disc can be calculated using the function and the width of the disc, and the volumes of the discs can be found by summing up these areas. This sum can be represented by a definite integral, which can be evaluated using numerical methods or analytically.
The choice of axis of revolution, either horizontal or vertical, will determine the appropriate formula for the area of each disc and the limits of integration for the definite integral. It is important to correctly orient the axis of revolution and choose the appropriate limits of integration to ensure that the correct volume is calculated.
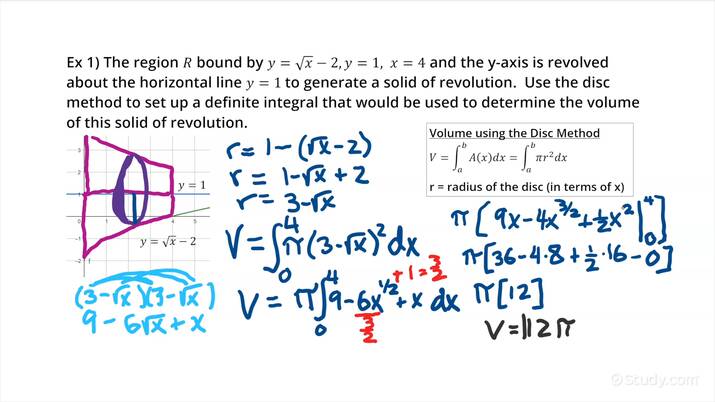
Source: Study
Overall, this topic is similar to topic 8.9 except we will be revolving our shape around other axes instead of just the x- or y-axis. This can get a bit confusing but we’ll simplify it for you with an example problem. 🔍
Example Problem: Finding the Volume Using the Disc Method Around Other Axes
To keep it simple, we’ll use the same y = √x curve from the example in topic 8.9. This time, however, we’ll revolve around the line y = 1 from x = 1 and x = 4. 🐪
If you rotate this region around the line y = 1, the cross sections will be circles with radii (√x) - 1. This is because our region is now shifted up one. When revolving around a line that is not the x- or y-axis, we must remember to take that into account when calculating the radius of our discs. With that, the area of each cross section will be π((√x) - 1)2. This can be simplified to π(x - 2√x + 1). Now we can integrate π(x - 2√x + 1) from x = 1 and x = 4 to get the volume.
Example 2
Suppose we have the function f(x) = x^2, and we want to find the volume of the solid of revolution obtained by rotating the region bounded by the x-axis and the graph of f(x) = x^2 around the vertical line x = 3. ✊
We can approximate the solid by a large number of thin circular discs, each with radius equal to the distance between the line x = 3 and the graph of f(x). The width of each disc is given by Δx, and the volume of each disc can be calculated as:
V = π * (f(x) - 3)^2 * Δx
The sum of the volumes of all the discs can be represented by a definite integral:
V = π * ∫_{a}^{b} (f(x) - 3)^2 dx
where a and b are the lower and upper limits of integration, respectively. In this case, since we are rotating the region bounded by the x-axis and the graph of f(x) = x^2, a = 0 and b = 3.
Therefore, the definite integral representing the volume of the solid of revolution is:
V = π * ∫_{0}^{3} (x^2 - 3)^2 dx
This integral can be evaluated using numerical methods or analytically, giving the volume of the solid. Note that this solution is only an approximation, and the accuracy of the solution will depend on the number of discs used and the width of each disc!
Browse Study Guides By Unit
👑Unit 1 – Limits & Continuity
🤓Unit 2 – Fundamentals of Differentiation
🤙🏽Unit 3 – Composite, Implicit, & Inverse Functions
👀Unit 4 – Contextual Applications of Differentiation
✨Unit 5 – Analytical Applications of Differentiation
🔥Unit 6 – Integration & Accumulation of Change
💎Unit 7 – Differential Equations
🐶Unit 8 – Applications of Integration
🦖Unit 9 – Parametric Equations, Polar Coordinates, & Vector-Valued Functions (BC Only)
♾Unit 10 – Infinite Sequences & Series (BC Only)
📚Study Tools
🤔Exam Skills

Fiveable
Resources
© 2025 Fiveable Inc. All rights reserved.