Dalia Savy
Jeremy Kiggundu
AP Chemistry 🧪
269 resourcesSee Units
When taking a look at the periodic table, you may wonder how scientists discovered all these numbers! As we discussed in the last section of this unit, an atom is made up of protons, neutrons, and electrons. Understanding these three subatomic particles and how they contribute to the makeup of the periodic table is significant.
Review of the Periodic Table ⚗️
On the periodic table, you can find the following information for each element:
- Element symbol: Each element is represented by a unique symbol, typically consisting of one or two letters.
- Atomic number: The atomic number of each element, found above the element symbol, represents the number of protons in the nucleus of the atom of that element. It is also the number of electrons in a neutral atom of that element, which we will discuss more later in this unit.
- Atomic mass: The atomic mass of an element is found below the element symbol and is typically expressed in atomic mass units (amu). The atomic mass is a representation of the number of protons in an atom of that element + the number of neutrons in an atom of that element. This will be our focus The atomic mass of elements represented on the periodic table will be our focus in this guide!
The Average Atomic Mass of an Element
Let's take a look at carbon on the periodic table that will be given to you during the AP exam:
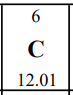
Here is what we know so far about carbon based on the periodic table:
- Carbon is represented by the element symbol "C."
- Carbon's atomic number is 6, which means one atom of carbon has 6 protons in its nucleus and 6 electrons orbiting its nucleus.
- Carbon's atomic mass looks to be 12.01, but how would this make sense? Since atomic mass = protons + neutrons, and we know we have 6 protons, that would give us 6.01 neutrons. As you may realize, this is impossible.
Instead of a nice "12" or "13" under Carbon, there's a really messy decimal of 12.01. If you take a look at other elements on the periodic table, you'll notice a similar trend. Hydrogen has an atomic mass of 1.008, and iron (Fe) has an atomic mass of 55.85.
This is all because the atomic masses that you're given on the periodic table are actually the average atomic masses of these elements. The average atomic mass of an element is the weighted average of the masses of the naturally occurring isotopes of that element, based on their relative abundances.
🎥 Watch Jacob Jeffries discuss the parts of the atom and the experiments scientists use to study them.
What are isotopes?
Isotopes are variants of an element. They have the same number of protons and electrons, but a different number of neutrons. This means that isotopes have the same atomic number (number of protons) but a different atomic mass (total number of protons and neutrons).
Therefore, the average atomic mass represents all of the isotopes of an atom, and how often they occur naturally in the environment. Let's break this down further. 🤔
Carbon's 3 Naturally Occurring Isotopes
There are three naturally occurring isotopes of carbon: carbon-12, carbon-13, and carbon-14.
- Carbon-12 is the most abundant isotope of carbon, making up about 98.9% of naturally occurring carbon. It has an atomic mass of 12 amu and is stable. Carbon-12 has 6 neutrons, which is calculated by taking the mass number of 12 and subtracting 6 protons.
- Carbon-13 is a less abundant isotope of carbon, making up about 1.1% of naturally occurring carbon. It has an atomic mass of 13 amu and is also stable. Carbon-13 has 7 neutrons: mass number of 13 - 6 protons
- Carbon-14 is a rare isotope of carbon, making up about 1 part per trillion of naturally occurring carbon. It has an atomic mass of 14 amu and is radioactive. Fun fact, carbon-14 is produced in the Earth's atmosphere through the interaction of cosmic rays with nitrogen atoms. 💥
- Also, since carbon-14 is found in small amounts in all living organisms, this is what is used when carbon dating and determining the age of organic materials discovered.
- Since there is barely any carbon-14 naturally occurring on Earth, we do not have to account for it when calculating the average atomic mass.
Calculating Carbon's Average Atomic Mass
So how do we calculate the average atomic mass of 12.01 knowing this information about carbon's naturally occurring isotopes? Here is what we know so far:
- Carbon's 2 main isotopes, Carbon-12 and Carbon-13, account for roughly 100% of the Carbon in nature.
- 98.9% of all carbon in nature is carbon-12, and the remaining 1.1% is carbon-13.
The average atomic mass of an element can be calculated using the following formula:
Average atomic mass = (abundance of isotope 1 x mass of isotope 1) + (abundance of isotope 2 x mass of isotope 2) + ... +
To calculate the average atomic mass of carbon from this data:
AAM = 0.989(12) + 0.011(13) = 12.01, which is the same as the number on the periodic table.
Let's see what would happen if we changed the numbers. Let's say now the chemist finds that only 75% of the carbon in nature was carbon-12 and 25% was carbon-13. Now, the AAM is:
AAM = 0.75(12) + 0.25(13) = 12.25
As you can see, the abundance of isotopes can have a significant effect on the average atomic mass of an element. If you are only given the mass numbers 12 and 13, as well as the average atomic mass, you can easily tell which isotope exists in greater amounts in nature. Since 12.01 is much closer to 12 than 13 is, Carbon-12 is clearly more abundant.
Always convert the % given to a decimal when calculating the average atomic mass.
Mass Spectroscopy 📊
Mass spectrometry is a technique used to measure the mass and relative abundance of ions in a sample. This technique produces a graph, called the mass spectrum, that allows us to identify different isotopes of an element and the relative abundance of each isotope in nature.
Mass Spectrum of Carbon ✏️
Looking at the mass spectrum of the element carbon, we can identify the isotopes of carbon as carbon-12 and carbon-13.
Image Courtesy of Professor Bensely
The fact that carbon-12 gives a significantly greater signal tells us that carbon-12 is much more abundant than carbon-13 in nature.
Putting Isotopes and Mass Spectrums Together
Let's put all of this information together and try to identify an element just from its mass spectrum. Here is an example:
The peaks below show a mass spectrum of element X. What is element X based on this spectrum? What is its average atomic mass?
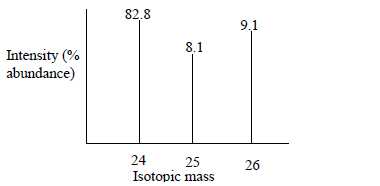
Image Courtesy of Kenyaplex
Without a calculator, you can easily tell that the average atomic mass is going to be somewhere between 24 and 25 since X-24 has the greatest % natural abundance. However, let's do some work! Here is what you should do when approaching this problem:
AAM = 0.828(24) + 0.081(25) + 0.091(26) = 24.263. This tells us that element X has an average atomic mass of approximately 24.3. When looking at the periodic table, we can identify this element as Magnesium:
Sample AP Question - 2007 Exam
Part a of question 2 on the AP Chemistry 2007 Exam (Form B) includes basic stoichiometry and advanced mass spectrum calculations. Here it is:
Question 2a: Part i
Instead of giving you the graph and asking you to solve for the average atomic mass, they are giving you the average atomic mass and asking you to solve for the percent abundance.
Don't worry! As long as you remember the basic formula, you can solve this problem:
AAM = (natural abundance)(mass) + ... + (natural abundance)(mass)
Now, just plug in what you know!
20.18 = (natural abundance)(19.99) + (natural abundance)(21.99)
One crucial piece of information that you have to remember for this question is that the percent abundances add up to 100%. This allows us to have x as one natural abundance and 1-x as the other, as you have probably done in previous algebra classes. Therefore, the setup for this problem is:
20.18 = (x)(19.99) + (1-x)(21.99)
Once you solve for x, you would get x = 0.905. Therefore, the percent abundance for Ne-20 is 90.5% and the percent abundance for Ne-22 is 9.5% (100%-90.5%).
Question 2a: Part ii
To recall from the last guide, you always want to put the value you know first when doing dimensional analysis. Well, how do you even know you have to do stoichiometry? This question is asking you to convert from grams to atoms which should give you a signal that dimensional analysis should be performed. Try this problem on your own first but here is the setup:
In the first conversion step, you are simply using the molar mass of neon on the periodic table. In the second conversion step, you are using the ratio of Ne to Ne-22, which is the percent abundance you found in part i. Then, you use Avogadro's number to convert to atoms and you get the answer of 3.558 x 10^22 Ne-22 atoms.
Mass Spectroscopy as a Laboratory Procedure
Mass Spectroscopy is more of a lesson that is geared towards the laboratory aspect of Chemistry🧪, but nonetheless, here’s an excellent lesson on Khan Academy which reviews mass spectroscopy:
🎥Watch: Khan Academy - Mass Spectroscopy
Browse Study Guides By Unit
⚛️Unit 1 – Atomic Structure & Properties
🤓Unit 2 – Molecular & Ionic Bonding
🌀Unit 3 – Intermolecular Forces & Properties
🧪Unit 4 – Chemical Reactions
👟Unit 5 – Kinetics
🔥Unit 6 – Thermodynamics
⚖️Unit 7 – Equilibrium
🍊Unit 8 – Acids & Bases
🔋Unit 9 – Applications of Thermodynamics
🧐Exam Skills
📚Study Tools

Fiveable
Resources
© 2025 Fiveable Inc. All rights reserved.