AP Stats Unit 4 FRQ Practice Prompt Answers & Feedback

FRQ Prompt

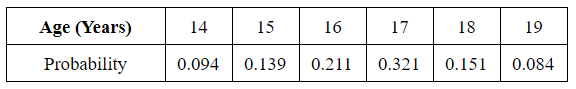
1. If a student-athlete is chosen from the district at random, what is the probability that they are at least 16 years old?
2. Calculate the expected age of a randomly-selected student-athlete.
3. If a random sample of 5 student-athletes is selected, what is the probability that 4 or 5 of the selected athletes are at least 16 years old?

Writing Samples and Feedback

Free Response Question Sample Submission 1

1. The probability that the student-athlete chosen from a random district is at least 16 years old is .767. (.211+.321+.151+.084
2. The expected age of a randomly-selected student-athlete is 16.548 years old. (14x.094)+(15x.139)+(16x.211)+(17x.321)+(18x.151)+(19x.084)
3. The probability that 4 or 5 of the selected athletes are at least 16 years old is .6686. (binompdf(5,.767,4)+binompdf(5,.767,5).

Your responses for all three parts have the correct calculations and answers (yay!). Be careful in #3 with the “calculator label”: you would not earn credit for the work as you showed it if this were the real AP exam (and your answer would be scored as “partial” credit). When doing binomial calculations, you have to make it clear what n and p are, which your response does not explicitly do (you can quite literally draw arrows with n/p or put “binompdf(n = 5, p = .767, X = 4)” to remedy this). It’s also good practice to write out “binomial” somewhere in your response. I typically encourage students to put a little “side work” on their paper: something like “binomial scenario: n = 5, p = .767, X = 4 or X = 5”… and then you’ve communicated everything you need to and can put down the results from your calculator.

Free Response Question Sample Submission 2

1. P(at least 16 years old) = P(16 years old) + P(17 years old) + P(18 years old) + P(19 years old) P(at least 16 years old) = 0.211+0.321+0.151+0.084 = 0.767. There is a 0.767 probability that if a student athlete is at least 16 years old if chosen from the district at random.
2. E(x) = mux = ∑xi * Pi = (140.094)+(150.139)+(160.211)+(170.321)+(180.151)+(190.084) = 16.548 years. The expected age of a randomly-selected student-athlete is 16.548 years.
3. Binomial Distribution Conditions:
   1. Binary - at least 16 or under 16, Independent - since you can only be in no more than one age group, the student-athletes’ ages are independent, Number of Trials = there are five students who are selected (n=5), Same Probability = probability of being at least 16 is the same for each student-athlete (p = 0.767)
   2. X = the number of student-athletes that are at least sixteen years old where the distribution of x is binomial with p = 0.767 and n = 5
   3. Using binomial cdf on TI Calculator with n = 5, p = 0.767, lower bound = 4, upper bound = 5, the calculated probability is 0.6686.
   4. There is a 0.6686 probability that 4 or 5 of the selected athletes are at least 16 years old if a random sample of 5 student-athletes is selected.

All of your answers are correct, with work shown. A small thing: on #3, when checking “independent”, it looks like you are making a reference to not being in more than one age group at a time… which would make the event mutually exclusive, not “independent.” Independence is when knowing the result of the first trial has no impact on future trials (which you check with your “same probability” statement). Independence can be assumed in this case because the small sample size (5) is much less than 10% of the population size (# of athletes in a “large suburban school district”), so we can assume independence even though we’re sampling w/o replacement.

Free Response Question Sample Submission 3

1. The probability that a randomly chosen student-athlete at least 16 years old is .767 (0.211+.321+.151+.084)
2. The expected age of a randomly-selected student is 16. To find that value I did: 14(.094)+15(.139)+16(.211)+17(.321)+18(.151)+19(.084)
3. The probability that 4 or 5 of the selected athletes are at least 16 years old is 66.8%. To find the value I first checked the binomial experiment requirements:
   1. Each trial has 2 possible outcomes
   2. There’s a fix number of trials (5)
   3. Outcomes are independent
   4. Probability of success is the same for each trial
   5. I then proceeded to calculate the probability by entering onto my calculator binompdf(5, .767,4) and binompdf(5,.767,5). After that I added the two values I got which ended up being .668.

Good work on showing your calculations on all parts. All calculations are done correctly; however it looks like in #2 you’ve rounded your answer to the whole number 16. It’s OK for expected value to a decimal or other number that isn’t technically possible for a single individual, because expected value represents a long-run average. That is, if we randomly select many players, the average age of a randomly selected player will be about 16.548 years old - and we should leave the “.548” attached.

Free Response Question Sample Submission 4

1. The probability that a student-athlete chosen from the district at random is at least 16 years old is: P(16)+ P(17) + P(18) + P(19)= 0.211+0.321+0.151+0.084= 0.767
2. Expected age of a randomly-selected student-athlete: (14 * 0.094) + (150.139)+ (160.211)+ (170.321) + (180.151) + (19*0.084)= 16.548 years old.
3. Conditions for a binomial experiment:
   1. Binary- There are 2 possible outcomes: At least 16 or under 16.
   2. Independent- The sample size of 5 students is less than 10% of the population (athletes in a large suburban school district).
   3. Number of Trials- There are a set number of trials (5)
   4. Same probability of success- Probability of being a student athlete that is at least 16 years old is 0.767 for all chosen athletes.
   5. Calculator: P(4 or 5 of the selected athletes are at least 16 years old) = [Binompdf(n=5, p=0.767, X=5)] + [Binompdf(n=5, p=0.767, X=4] = 0.6686

Your answers to #1 and #2 are correct with work shown. For #3, you’ve included more than you would typically need for a situation like this. On most rubrics, simply identifying the scenario as “binomial” will work (without citing all of the conditions) - as long as you clearly label the values of n, p, and X that are involved.