9.11 MC Answers and Review
5 min readβ’december 31, 2021
Dalia Savy
AP Calculus AB/BCΒ βΎοΈ
279Β resourcesSee Units
Answers and Review for Multiple Choice Practice on Parametric Equations, Polar Coordinates & Vector-Valued Functions
Parametric graph created in Desmos.
βSTOP!β Before you look at the answers make sure you gave this practice quiz a try so you can assess your understanding of the concepts covered in unit 9. Click here for the practice questions: AP Calculus Unit 9 Multiple Choice Questions.
Facts about the test: Both the AP Calculus AB and BC exams have 45 multiple-choice questions and you will be given 1 hour and 45 minutes to complete the section. This means it should take you about 35 minutes to complete 15 questions.
*The following questions were not written by CollegeBoard and although they cover information outlined in the AP Calculus AB/BC Course and Exam Description, the formatting on the exam may be different.
1. Which of the following is the derivative formula for differentiating a parametric equation?
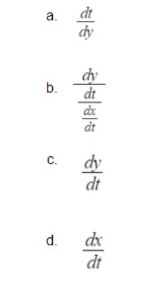
Answer: The answer is B. The derivative formula for a parametric equation is when you differentiate y and x separately in terms of t.
π Study AP Calculus, Unit 9.1: Defining and Differentiating Parametric Equations
2. Given x = 2βt and y = 3t^2 -2t. Find dy/dx. Evaluate at t=1.
A. -4
B. -2
C. 4
D. 0
Answer: Take the derivative of each function separately. Then plugin 1.
π Study AP Calculus, Unit 9.1: Defining and Differentiating Parametric Equations
3. What is the second derivative formula for a parametric equation?
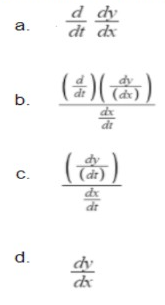
Answer: The answer is B. The numerator is the second derivative of the first derivative and the denominator is the first derivative of the x function.
π Study AP Calculus, Unit 9.2: Second Derivatives of Parametric Equations
4. Find all the points of horizontal and vertical tangency given x = t^2 + t and y = t^3 - 3t + 5.
A. t = -1 and -1/2Β
B. t = 1 and -1/2
C. t = 1 and -1
D. t = 1, -1, and -1/2
Answer: Take the first derivative. Set the numerator equal to zero for the horizontal tangent. Set the denominator equal to zero for the vertical tangent.
π Study AP Calculus, Unit 9.1: Defining and Differentiating Parametric Equations
5. What is the arc length formula for a parametric equation?
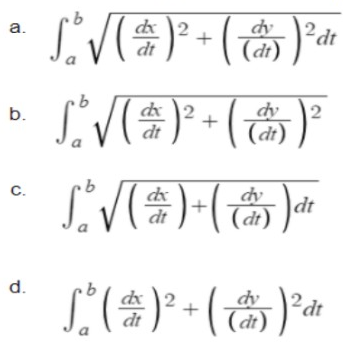
Answer: The answer is A. You must remember to square each of the functions and take the square root.
π Study AP Calculus, Unit 9.3: Finding Arc Lengths of Curves Given by Parametric Equations
6. What is the distance traveled by the object from t=1 to t=7 if the functions are dx/dt = tsin(t) and dy/dt=cos(t^2)
A. 15
B. 14.802
C. 13.015
D. 16
Answer: Plug into a graphing calculator!
π Study AP Calculus, Unit 9.3: Finding Arc Lengths of Curves Given by Parametric Equations
7. To find the speed of the particle, you take the absolute value of theβ¦
A. position vector.Β
B. velocity vector.
C. acceleration vector.
D. displacement vector.
Answer: This should be the absolute value of the velocity vector. The speed can also sometimes be referred to also as the magnitude vector.
π Study AP Calculus, Unit 9.6: Solving Motion Problems Using Parametric and Vector-Valued Functions
8. When converting between polar and rectangular coordinates, you can use the following formulas to help you find x and y:
A. x = rcosΞΈ and y = rsinΞΈ
B. y = rcosΞΈ and x = rsinΞΈ
C. y=cosΞΈ and x = sinΞΈ
D. x = cosΞΈ and y = sinΞΈ
Answer: The equation will be A due to the connection to the unit circle of cosine being x and sine being represented by y.
π Study AP Calculus, Unit 9.7: Defining Polar Coordinates and Differentiating in Polar Form
9. The formula to find the derivative of a polar function is:
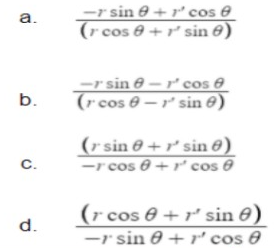
Answer: The answer is D since you are using the product rule of x=rcostheta and y=rsintheta.
π Study AP Calculus, Unit 9.7: Defining Polar Coordinates and Differentiating in Polar Form
10. What is the area formula for one polar curve?
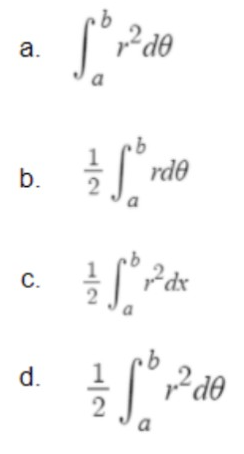
Answer: The answer is D as you need to square the radius and the function is in terms of theta.
π Study AP Calculus, Unit 9.8: Find the Area of a Polar Region or the Area Bounded by a Single Polar Curve
11. Find the area bounded by the graph of r = 3 + 3sinΞΈ.
A. 42
B. 42.412
C. 21
D. 21.206
Answer: Remember to half the integral!
π Study AP Calculus, Unit 9.8: Find the Area of a Polar Region or the Area Bounded by a Single Polar Curve
12. What is the formula for the polar area between two curves?
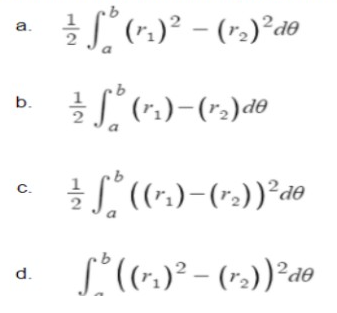
Answer: The answer is A. Remember to square each function when taking the integral.
π Study AP Calculus, Unit 9.9: Finding the Area of the Region Bounded by Two Polar Curves
13. Find the area inside the smaller loop of the limacon r = 2cosΞΈ + 1.
A. 1
B. 0.6
C. 0.544
D. 0.78
Answer: Find the points of intersection first which will be 4pi/3 and 2pi/3 then take the integral of this.
π Study AP Calculus, Unit 9.9: Finding the Area of the Region Bounded by Two Polar Curves
14. Determine the area that is inside r = 3 + 2sinΞΈ and outside r = 2.
A. 23
B. 24
C. 24.187
D. 25
Answer: Set the two functions equal to find the points of intersection. From there then find the integral.
π Study AP Calculus, Unit 9.9: Finding the Area of the Region Bounded by Two Polar Curves
15. What is the arc length formula of a polar curve?
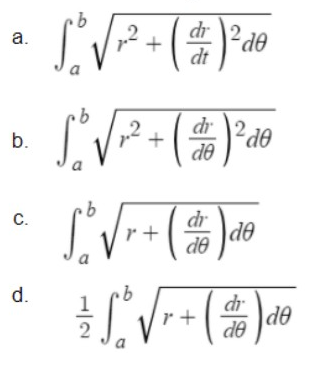
Answer: The answer is B. Both the derivative and the radius must be squared. You are differentiating in terms of theta.
π Study AP Calculus, Unit 9.3: Finding Arc Lengths of Curves Given by Parametric Equations
What can we help you do now?
πStudy for Unit 10
π¦Jump to AP Calculus Unit 10 Multiple Choice Questions
π€Connect with other students studying AP Calculus with Hours
Browse Study Guides By Unit
πUnit 1 β Limits & Continuity
π€Unit 2 β Fundamentals of Differentiation
π€π½Unit 3 β Composite, Implicit, & Inverse Functions
πUnit 4 β Contextual Applications of Differentiation
β¨Unit 5 β Analytical Applications of Differentiation
π₯Unit 6 β Integration & Accumulation of Change
πUnit 7 β Differential Equations
πΆUnit 8 β Applications of Integration
π¦Unit 9 β Parametric Equations, Polar Coordinates, & Vector-Valued Functions (BC Only)
βΎUnit 10 β Infinite Sequences & Series (BC Only)
π§Multiple Choice Questions (MCQ)
βοΈFree Response Questions (FRQ)
πBig Reviews: Finals & Exam Prep
Fiveable
Resources
Β© 2023 Fiveable Inc. All rights reserved.