5.9 Connecting a Function, Its First Derivative, and its Second Derivative
6 min read•february 15, 2024
B
Beth
AP Calculus AB/BC ♾️
279 resourcesSee Units
Overview
So, you're a pro at sketching the function and its derivative now, right? Well, now what? Your hard work wasn't for nothing; a function's derivative can be helpful in understanding behavior in the actual function and vice versa. Key features of the graphs of f, f', and f" are related to one another. You can use the graphs to justify conclusions about your functions.
Interpreting the graph of the function
Here's what you need to know:
- when a function is increasing, you know that the first derivative will be positive
- when a function is decreasing, the first derivative will be negative (the points in that range will be below 0)
- when a function is concave up, the second derivative is positive
- when a function is concave down, the second derivative is negative

Let's say you have this graph of function g(x)
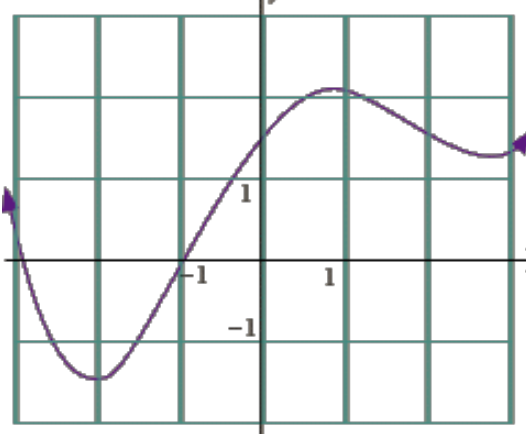
Now, let's describe the first derivative based on this graph:
- From (-∞,-2), the graph of g(x) is decreasing, therefore the graph of g'(x) over (-∞,-2) is negative
- From (-2,0.85), the graph of g(x) is increasing, therefore the graph of g'(x) over (-2,0.85) is positive
- From (0.85, 2.8), the graph of g(x) is decreasing, therefore the graph of g'(x) over (0.85, 2.8) is negative
- From (2.8,∞), the graph of g(x) is increasing, therefore the graph of g'(x) over (2.8,∞) is positive
We can also describe the second derivative based on the concavity of the graph (I am going to estimate the inflection point, but you can always find the exact, if you have the equation, using a calculator!)
- From (-∞,-0.5), the graph of g(x) is concave up, therefore the graph of g"(x) is positive, AND g'(x) is increasing
- From (-0.5, 1.5), the graph of g(x) is concave down, therefore the graph of g"(x) is negative, AND g'(x) is decreasing
- From (1.5, ∞), the graph of g(x) is concave up, therefore the graph of g"(x) is positive, AND g'(x) is increasing
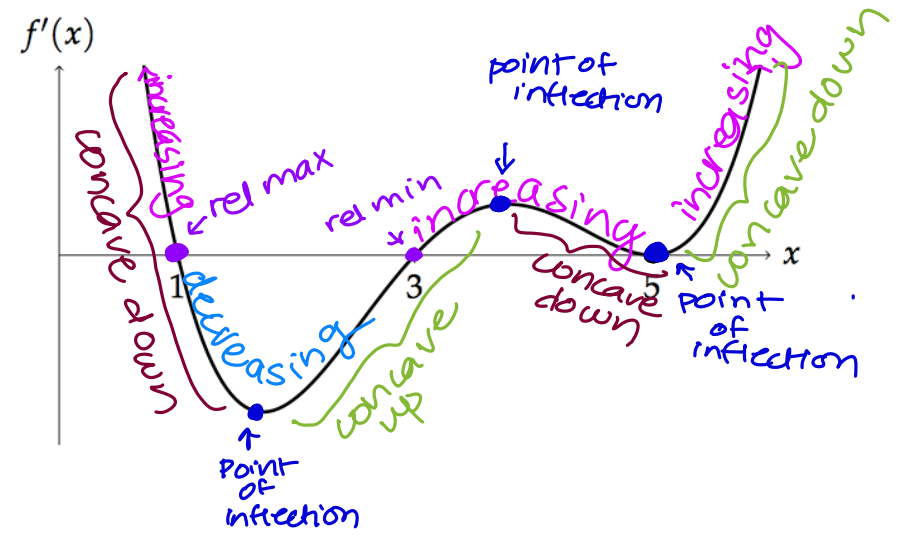
Based on where the graph of the function changes direction and concavity, we can also interpret maximums, minimums, x-intercepts, and points of inflection of the graphs of the first and second derivatives.
- If f(x) has a relative minimum (the graph changes from decreasing to increasing), then the graph of f'(x) will change from negative to positive at that point
- If f(x) has a relative maximum (the graph changes from increasing to decreasing), then the graph of f'(x) will change from positive to negative at that point
- If f(x) has a point of inflection changing from concave up to concave down, then the graph of f'(x) will have a relative maximum AND f"(x) will change from positive to negative at that point
- If f(x) has a point of inflection changing from concave down to concave up, then the graph of f'(x) will have a relative minimum AND f"(x) will change from negative to positive at that point
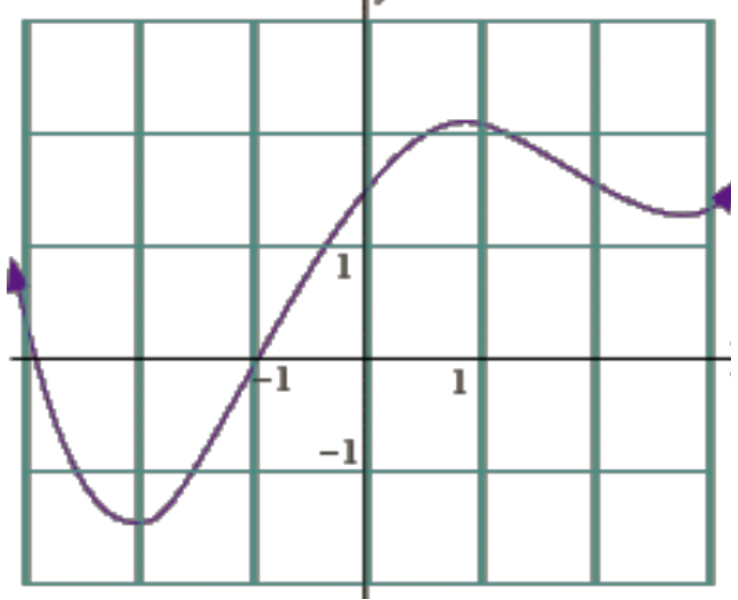
Let's continue to use the graph of g(x) to interpret relative maximums, relative minimums, points of inflection, and x-intercepts:
- at x=-2, f(x) has a relative minimum, therefore the graph of f'(x) will change from negative to positive at x=-2
- at x=0.85, f(x) has a relative maximum, therefore the graph of f'(x) will change from positive to negative at x=0.85
- at x=2.8, f(x) has a relative minimum, therefore the graph of f'(x) will change from negative to positive at x=2.8
- at x=-0.5, f(x) has a point of inflection changing from concave up to concave down, therefore f'(x) will have a relative maximum and f"(x) will change from positive to negative at x=-0.5
- at x=1.5, g(x) has a point of inflection changing from concave down to concave up, therefore g'(x) will have a relative minimum and g"(x) will change from negative to positive at x=1.5
After interpreting the graph of the function, we can sketch a graph of the first derivative or even the second derivative. g'(x) would look something like this:
In this graph, you can note each of the features we described by analyzing the graph of the function.
Interpreting the graph of the first derivative
Interpreting the graph of the first derivative
Now that we've got the hang of interpreting the graph of the function, let's reverse the order! Just as we can get information about the graph of the derivative from the graph of the function, we can get information about the graph of the function from the graph of the derivative. You basically just go backwards from what we already described.
- If f'(x) is positive, then the graph of f(x) is increasing
- If f'(x) is negative, then the graph of f(x) is decreasing
- If f'(x) is decreasing, then the graph of f(x) is concave down
- If f'(x) is increasing, then the graph of f(x) is concave up
- If f'(x) changes from positive to negative at x=k, then f(x) has a relative maximum at x=k
- If f'(x) changes from negative to positive at x=k, then f(x) has a relative minimum at x=k
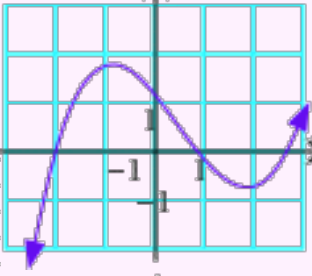
Let's practice describing f(x) using the graph of f'(x):
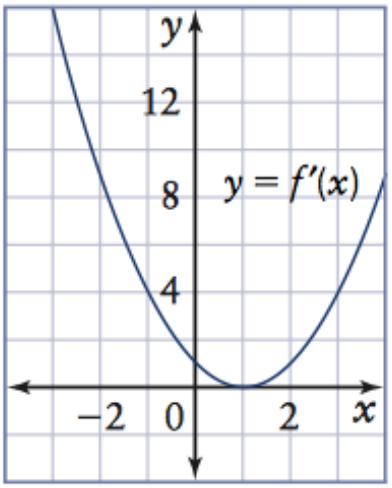
- f'(x) is always positive, therefore f(x) is always increasing
- from (-∞,1), f'(x) is decreasing, therefore f(x) is concave down
- from (1,∞), f'(x) is increasing, therefore f(x) is concave up
- at x=1, f'(x) changes from decreasing to increasing, therefore f(x) has a point of inflection at x=1
So here is an approximation of what the graph of f(x) would look like based on the characteristics we described by analyzing the first derivative:
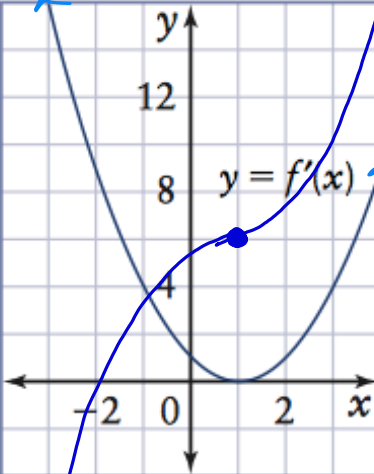
Common mistakes
- Misconception: The first derivative is always positive when the function is increasing > The first derivative can be positive even when the function is decreasing, as long as the rate of decrease is slowing down. Similarly, the first derivative can be negative even when the function is increasing, as long as the rate of increase is slowing down.
- Misconception: The first derivative is always negative when the function is decreasing > The first derivative can be negative even when the function is increasing, as long as the rate of increase is accelerating. Similarly, the first derivative can be positive even when the function is decreasing, as long as the rate of decrease is accelerating.
- Misconception: The first derivative is always zero at a local maximum or minimum > The first derivative is zero at a local maximum or minimum only if it is a flat maximum or minimum. If the maximum or minimum is "pointy," the first derivative will be non-zero at that point.
- Misconception: The first derivative is always positive at a local minimum > The first derivative can be negative at a local minimum if the minimum is "pointy."
- Misconception: The first derivative is always negative at a local maximum > The first derivative can be positive at a local maximum if the maximum is "pointy."
- Misconception: The graph of the first derivative is always the slope of the original function > The graph of the first derivative gives the rate of change of the original function at each point, but it is not the same as the slope of the original function.
- Misconception: The graph of the first derivative tells you the shape of the original function > The graph of the first derivative gives information about the rate of change of the original function, but it does not necessarily tell you the shape of the original function.
Browse Study Guides By Unit
👑Unit 1 – Limits & Continuity
🤓Unit 2 – Fundamentals of Differentiation
🤙🏽Unit 3 – Composite, Implicit, & Inverse Functions
👀Unit 4 – Contextual Applications of Differentiation
✨Unit 5 – Analytical Applications of Differentiation
🔥Unit 6 – Integration & Accumulation of Change
💎Unit 7 – Differential Equations
🐶Unit 8 – Applications of Integration
🦖Unit 9 – Parametric Equations, Polar Coordinates, & Vector-Valued Functions (BC Only)
♾Unit 10 – Infinite Sequences & Series (BC Only)
📚Study Tools
🤔Exam Skills

Fiveable
Resources
© 2025 Fiveable Inc. All rights reserved.